Back to: Mathematics Primary 4
HELLO, WELCOME BACK TO CLASS
Profit and Loss formula is used in mathematics to determine the price of a commodity in the market and understand how profitable a business is. Every product has a cost price and selling price. Based on the values of these prices, we can calculate the profit gained or the loss incurred for a particular product. The important terms covered here are cost price, fixed, variable and semi-variable cost, selling price, marked price, list price, margin, etc. Also, we will learn the profit and loss percentage methods. For example, for a shopkeeper, if the value of selling price is more than the cost price of a commodity, then it is a profit and if the cost price is more than the selling price, it becomes a loss. Here, in this article, we will discuss profit as well as loss concepts along with tricks to solve problems based on it.
Profit and Loss Basic Concepts
Profit (P): The amount gained by selling a product with more than its cost price. When an article or product is sold for more than what it costs, we say that there is a ‘profit’ or gain. Put simply, Profit (P) is the excess of selling price over cost price. Another name for profit is GAIN
Profit = Selling Price – Cost Price
Loss (L): The amount the seller incurs after selling the product less than its cost price, is mentioned as a loss. When an article or product is sold for less than what it costs, we say that there is a ‘loss’. Put simply, Loss (L) is the excess of cost price over selling price.
Loss = Cost Price – Selling Price
Cost Price (C.P.): The amount paid for a product or commodity to purchase it is called a cost price. Also, denoted as CP. This cost price is further classified into two different categories:
- Fixed Cost: The fixed cost is constant, it doesn’t vary under any circumstances
- Variable Cost: It could vary depending as per the number of units
Cost Price = Selling Price – Profit or, Selling Price + Loss
Selling Price (S.P.): The price or amount for which the product or article is sold is called Selling Price. It is usually denoted as (S.P.) Also, sometimes called a sales price.
Selling Price = Cost Price + Profit or, Cost Price – Loss
Break even (BE) is a situation where is no profit or loss. That is, the cost price is equal to selling price When the selling price is equal to the cost price, then there is neither profit nor loss.
Profit or Loss per cent = 
Examples
Ali buys a watch for $54 and sold it for $71. What is his profit or loss?
Cp=$54
Sp=$71
P=sp-cp
P= $71-$54=$17
Example 2:
Ada buys a spoon for #5.50 and sells it for #5.25. what is her profit or loss
Cost price =#5.50
Selling price = 5.25
Loss=0.25k
Mr. Smith bought a book for $ 85 and sold it for sold it for $ 115. Find his profit or loss percent.
Solution:
Cost Price (CP) = $ 85;
Selling Price (SP) = $ 115
Since SP > CP,
Therefore, Mr. Smith makes a profit.
Profit = Selling Price (SP) – Cost Price (CP)
= 115 – 85
= $ 30
Therefore, profit % = (Profit/Cost Price) × 100
= (30/85) x 100
= 35.29 %
Answers: 35.29 %
Mr. Brown bought a TV for $ 5800 and sold it for sold it for $ 7000. Find his profit or loss percent.
Solution:
Cost Price (CP) = $ 5800;
Selling Price (SP) = $ 7000
Since SP > CP,
Therefore, Mr. Brown makes a profit.
Profit = Selling Price (SP) – Cost Price (CP)
= 7000 – 5800
= $ 1200
Therefore, profit % = (Profit/Cost Price) × 100
= (1200/5800) x 100
= 20.69 %
Answers: 20.69 %
Robert bought pencils for $ 150.As they were of bad quality, he had to sell them for $ 127. Find his loss or gain percent.
Solution:
Cost Price (CP) = $ 150,
Selling Price (SP) = $ 127
Since SP < CP,
Therefore, Robert suffers a loss.
Loss = Cost Price (CP) – Selling Price (SP)
= 150 – 127
= $ 23
Therefore, loss % = (Loss/CP) × 100
= (23/150) × 100
= 15.33%
Answers: 15.33 %
4. Jack bought a pairs of shirt for $ 125 and sold them for $ 108. Find his loss or gain percent.
Solution:
Cost Price (CP) = $ 125,
Selling Price (SP) = $ 108
Since SP < CP,
Therefore, Jack suffers a loss.
Loss = Cost Price (CP) – Selling Price (SP)
= 125 – 108
= $ 17
Therefore, loss % = (Loss/CP) × 100
= (17/125) × 100
= 13.6 %
Answers: 13.6 %
Find Profit or Loss Percent.
Example 1: What is the profit per cent if a table bought for $65 is sold for $70?
Solution: A table is bought for and sold for $70.
Total profit 
Profit % 
Example 1: A man bought a T.V. set for and he sold it at a profit of . Find the selling price.
Solution: Let the cost price be
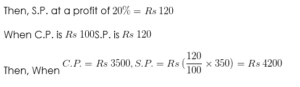
Alternative Method:
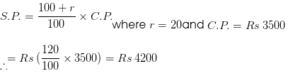
Example 2: A man buys a cycle for and sells it at a loss of . Find the selling price of the cycle.
Solution: Let the C.P. be
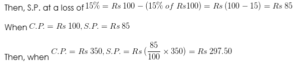
Alternative Method:
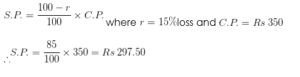
Type 3 : Find Cost Price.
Example 1: Find the cost price of an article which is sold at a profit of for .
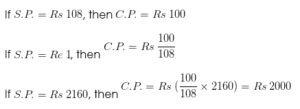
Alternative Method:
A few harder problems on profit and loss:
Example 1: By selling a plot of land for a person loses . At what price should he sell it so as to gain ?
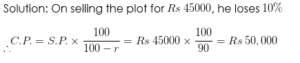
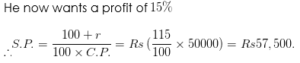
Example 2: A man sells two watches at each. On one he gains and on the other he loses . What is his gain or loss per cent on the whole transaction?
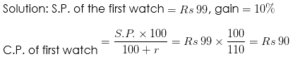
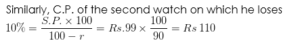
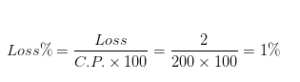
Discount
Marked Price: The price printed on an article or on a tag tied to it or the advertised price or the listed price is called the marked price, or, M.P. of the article.
Sometimes to dispose of the old, damaged or perishable goods the retailers offer these goods at reduced prices. The retailers also reduce prices to increase the sale by reducing the marked prices of the articles. The amount deducted from the original marked prices is called ‘Retailer’s discount’ or simply ‘retail discount’ which is generally expressed as per cent or a fraction of the marked or original price.
Net Price (Selling Price): The price of an article after deducting discount from the marked price is called the net price of the article.
NOTE: Discount is always calculated on the marked price.
In solving the problems on discount, the following formula are generally used:
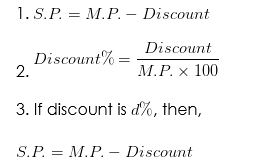
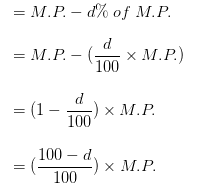
Example 1: The marked price of a pair of shoes is . The shopkeeper allows an off season discount of on it. Calculate – i) the discount and ii) the selling price.
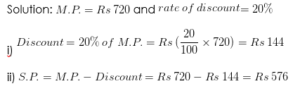
Example 2: The marked price of an article is marked above the C.P. and then it is sold at a discount of . What is the net gain per cent?
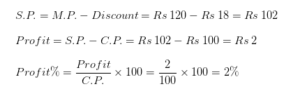
Quiz
- A cloth merchant on selling of cloth obtains a profit equal to the selling price of of cloth. Find his profit per cent.
- An article was sold at a loss of 4%. Had it been sold for #26 more, there would have been a profit of 9%. Find the cost price.
- A shopkeeper allows 25% off on the marked price of an article and still gets a profit of 20%. What is the marked price of the article when it’s cost price is #300?
- By selling bananas, a vendor loses the selling price of bananas. Find his loss per cent.
- A tradesman allows a discount of 5% on the marked price of goods. How much above the cost price must he mark his goods to make a profit of 14%
Get more class notes, videos, homework help, exam practice on Android [DOWNLOAD]
Get more class notes, videos, homework help, exam practice on iPhone [DOWNLOAD]
NICE TEACHING
I