Mean Deviation, Variance and Standard Deviation

Back to: MATHEMATICS SS1

 

Welcome to class! 

In today’s class, we will be talking about mean deviation, variance and standard deviation. Enjoy the class!

Mean Deviation, Variance and Standard Deviation

Mean deviation

Example:

The Mean Deviation of 3, 6, 6, 7, 8, 11, 15, 16

Step 1: Find the mean

Mean = 3 + 6 + 6 + 7 + 8 + 11 + 15 + 168 = 728 = 9

Step 2: Find the distance of each value from that mean

Value	Distance from 9
3	6
6	3
6	3
7	2
8	1
11	2
15	6
16	7

Find the mean of those distances:

Mean Deviation = 6 + 3 + 3 + 2 + 1 + 2 + 6 + 78 = 308 = 3.75

So, the mean = 9, and the mean deviation = 3.75

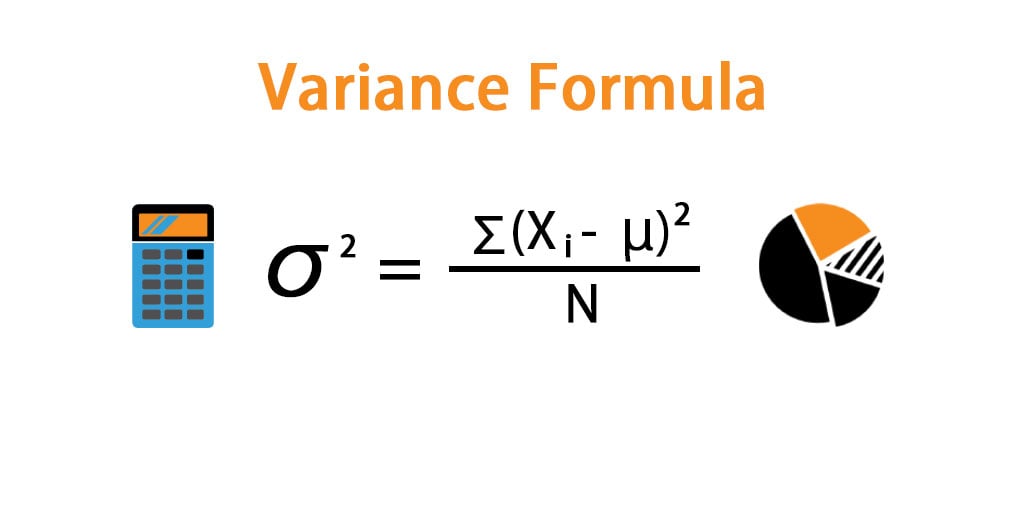
Variance

Let a population consist of nn elements, {x1; x2; …; xn}. Write the mean of the data as x¯

The variance of the data is the average squared distance between the mean and each data value.

σ2=∑ni=(1(xi−x¯¯¯)2)/n

You flip a coin 100100 times and it lands on heads 4444 times. You then use the same coin and do another 100100 flips. This time in lands on heads 4949 times. You repeat this experiment a total of 1010 times and get the following results for the number of heads.

{44;49;52;62;53;48;54;49;46;51}

Compute the mean and variance of this data set.

Compute the mean

The formula for the mean is

x¯¯¯=∑ni=1xin

In this case, we sum the data and divide by 10 to get x¯¯=50,

Compute the variance

The formula for the variance is

σ2=∑ni=1(xi−x¯¯¯)2/n

xi	44	49	52	62	53	48	54	49	46	51
xi−x¯¯¯	−6,8	−1,8	1,2	11,2	2,2	−2,8	3,2	−1,8	−4,8−4,8	0,20,2
(xi−x¯¯¯)2	46,24	3,24	1,44	125,44	4,84	7,84	10,24	3,24	23,0423,04	0,040,04

We first subtract the mean from each datum and then square the result.

The variance is the sum of the last row in this table divided by 10, so σ2=22,56

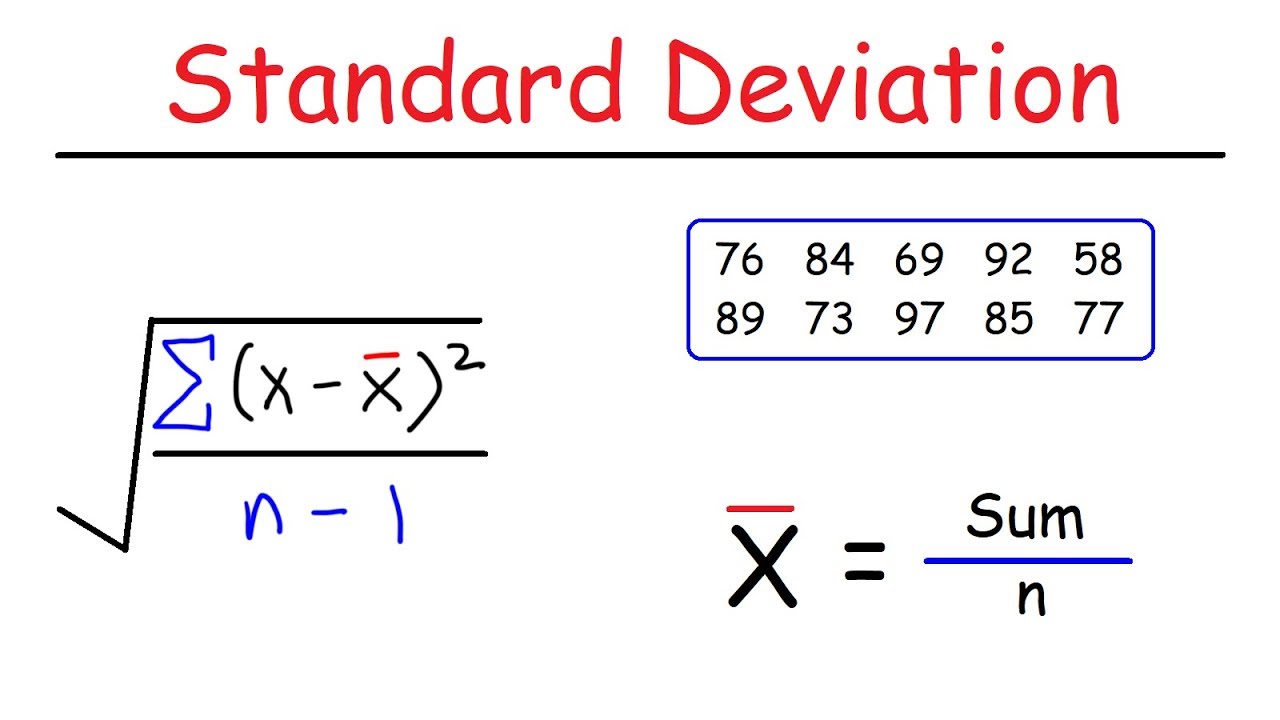
Standard deviation

Let a population consist of n elements, {x1; x2; …; xn} with a mean of x¯. The standard deviation of the data is

σ=√∑ni=1(xi−x¯¯¯)2 / n

What is the variance and standard deviation of the possibilities associated with rolling a fair die?

Determine all the possible outcomes

When rolling a fair die, the sample space consists of 66 outcomes. The data set is therefore x={1;2;3;4;5;6} and n=6

Calculate the mean

The mean is:

x¯¯¯ = 1/6 (1+2+3+4+5+6)

=3,5

Calculate the variance

The variance is:

σ2=∑(x−x¯¯¯)2n

=1/6 (6,25+2,25+0,25+0,25+2,25+6,25)

=2,917

Calculate the standard deviation

The standard deviation is:

σ=√2,917

=1,708

 

We hope you enjoyed the class.

Should you have any further question, feel free to ask in the comment section below and trust us to respond as soon as possible.

School Owner? Looking for ready-made content and tools to save time and grow easily? Book a free demo session now

Get more class notes, videos, homework help, exam practice on Android [DOWNLOAD]

Get more class notes, videos, homework help, exam practice on iPhone [DOWNLOAD]