CONSTRUCTION

Welcome to class!

In today’s class, we will be talking about construction. Enjoy the class!

Construction of parallel and perpendicular lines
Bisection of a given line segment
Construction of angles 90 and 60 degrees

Construction of Parallel and Perpendicular Lines:

In this section, you will learn how to construct parallel and perpendicular lines.

Parallel Lines:

Parallel lines are the lines which will never intersect and the perpendicular distance between them will be the same at everywhere.

Perpendicular Lines:

The two lines which have the angle of inclination 90° at the point of intersection are called as perpendicular lines.

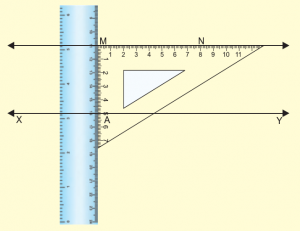
Construction of Parallel Lines – Example

Using a set square and a ruler draw a line parallel to a given line through a point at a distance of 5cm above it.

Step 1:

(i) Draw a line XY using a ruler and mark a point A on it.

(ii) Draw AM = 5cm with the help of a set square.

Step 2:

Place the set square on the line segment XY.

(i) Place the set scale as shown in the figure.

Step 3:

(i) Pressing tightly the ruler, slide the set square along the ruler till the edge of the set square touches the point M.

(ii) Through M, draw a line MN along the edge.

(iii) MN is the required line parallel to XY through M.

Construction of Perpendicular Lines – Examples

Example 1:

Using a set square and a ruler, draw a line perpendicular to a given line at a point on it.

Solution:

Step 1:

(i) Draw a line AB with the help of a ruler.

(ii) Mark a point P on it.

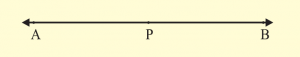
Step 2:

(i) Place a ruler on the line AB

(ii) Place one edge of a set square containing the right angle along with the given line AB as shown in the figure.

Step 3:

(i) Pressing the ruler tightly with the left hand, slide the set square along the ruler till the edge of the set square touches the point P.

(ii) Through P, draw a line PQ along the edge.

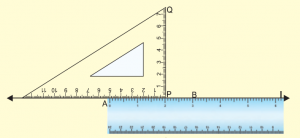
Step 4:

PQ is the required line perpendicular to AB. Measure and check if m<APQ = m<BPQ = 90°

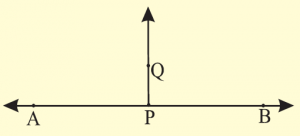
Example 2:

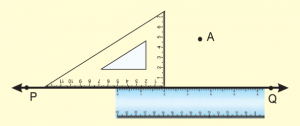
Using a set square and a ruler, draw a line perpendicular to the given line through a point above it.

Solution:

Step 1:

(i) Draw a line PQ using a ruler

(ii) Mark a point A above the given line

Step 2:

(i) Place the ruler on the line PQ

(ii) Place one edge of a set square containing the right angle along the given line PQ as shown in the figure.

Step 3:

(i) Pressing tightly the ruler with the left hand, slide the set square along the ruler till the edge of the set square touches the point A

(ii) Through A draw a line AO along the edge.

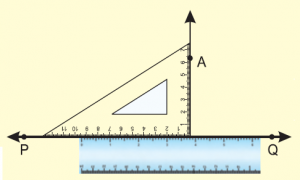
Step 4:

(i) AO is the required line perpendicular to PQ.

(ii) Measure and check: m<POA = <mQOA = 90°

These two angles (140° and 40°) are Supplementary Angles because they add up to 180°:

But the angles don’t have to be together.

These two are supplementary because 60° + 120° = 180°

They don’t have to be next to each other, just so long as the total is 180 degrees.

Examples 3:

60° and 120° are supplementary angles.
93° and 87° are supplementary angles.

Two Angles are Complementary when they add up to 90 degrees (a Right Angle).

They don’t have to be next to each other, just so long as the total is 90 degrees.

Examples 4:

60° and 30° are complementary angles.
5° and 85° are complementary angles.

These two angles (40° and 50°) are Complementary Angles because they add up to 90°:

But the angles don’t have to be together.

These two are complementary because 27° + 63° = 90°

In our next class, we will be talking about Statistics. We hope you enjoyed the class.

Should you have any further question, feel free to ask in the comment section below and trust us to respond as soon as possible.

Ready to make school management and growth easy? Book your free onboarding session now

Get more class notes, videos, homework help, exam practice on Android [DOWNLOAD]

Get more class notes, videos, homework help, exam practice on iPhone [DOWNLOAD]

CONSTRUCTION