Back to: Mathematics Primary 3
Fractions that represent the same amount are called equivalent fractions.
Example 2 : Find an equivalent fraction for 1/3.
One Way: Use fraction bars

Another way : Use Multiplication
Multiply the numerator and the denominator by 2 (You can as well use any whole number you wish except zero)
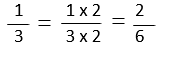
How to Order Fractions?
Ordering fraction simply mean arranging fraction either from the smallest to the largest (ascending order) or largest to smallest (descending order).
There are two common methods or ordering fractions.
These are:
- Using a common denominator.
- Changing fraction to decimals and then ordering.
Ordering Fractions using Common Denominator
Fractions can be compared and ordered by determining their equivalent fractions with the common denominator. Common denominators are created by using common multiples of the two numbers, for example 24 is the least common multiple of 8 and 12.
8 x 3 = 24
12 x 2 = 24
Yet 8 and 12 have several other common multiples, however, 24 is the lowest.

Since the fractions have different denominators;
Step 1: Multiply the denominators; (4 x 5) = 20
Step 2: Working with the first fraction 3/4 , divide the 20 by 4 = 5
Step 3: Multiply the numerator of fraction 3/4 by the 5, i.e 3 x 5 = 15
Step 4: Working with the other fraction 2/5 , divide the 20 by 5 = 4
Step 5: Multiply the numerator of fraction 2/5 by 4, i.e 2 x 4 = 8
It is easy to see that 15 is greater than 8.
So, 3 / 2/
(>)
4 5
Example 6 : Arrange 1/5, 1/3, 2/3 in descending order.
1/5, 1/3, 2/3
The LCM of the denominators (5, 3 and 3) is 15
So, we have 1/5, 1/3, 2/3
3, 5, 10
15
In Descending order, we have 2/3, 1/3, 1/5
Addition of Like Fractions
Like Fractions are fractions with the same denominator.
Example 1: Pablo spent 3/5 of an hour on a jigsaw puzzle. Conrad spent 1/5 of an hour on the puzzle. How much time did they spend working on the puzzle in all?
You need to add 3/5 and 1/5.
Note this: To add fractions with the same denominators, add the numerators and keep the same denominator.
3/5 + 1/5
3 + 1/ 5 4
Example 2: Liliana jogged 5/8 of a mile on Monday and 3/8 of a mile on Tuesday. How much farther did she jog on Monday?
You need to subtract 5/8 and 3/8.
5/8 – 3/8
5 – 3 /8= 2 /8 Subtract numerators.
Keep the same denominators
2 ÷ 2 / 8 ÷ 2 = 1 /4 Write the answer in simplest form.
